Why Vangel Vesovski is Wrong

(c) 2009 by Barton Paul Levenson

Vangel Vesovski is a Libertarian poster on the amazon.com boards who insists that anthropogenic global warming (AGW) theory is "a hoax." One of his favorite talking points, which he repeats again and again, is that NASA GISS temperature measurements are unreliable, as are any measurements but those of the satellites, and that the satellite data shows a cooling trend since 1998. In fact, of the two major satellite temperature series, RSS and UAH, VV denigrates the former in favor of the latter. The reason why is pretty obvious; UAH shows the lowest rising temperature trend. It's an outlier, but it's an outlier congenial to the denier position.

There are all kinds of things wrong with his talking point. I'll ignore the massive evidence for rising temperature, which is by no means confined to NASA GISS temperature station measurements. Let's assume UAH is the only legitimate temperature data and examine some of the problems which still remain with VV's thesis.

1. The World Meteorological Organization defines climate as mean regional or global weather over a period of 30 years or more. VV is isolating 11 years of data here.
2. He picked 1998 because it was a local high point in the curve of temperature against time. Temperature jogs up and down. If you pick out a small portion of the chart and measure it starting from a high point, you're likely to get an apparent downward trend. This is called "cherry picking," and will get you a flunking grade in any introductory data analysis course. You can't just take out a portion of the data that seems to show what you want; you have to use all the data.
3. A trend has to be statistically significant (see point 1). Even if the regression line were down from 1998 to 2008, it wouldn't prove that the trend had changed unless the slope was significantly downward as measured by the t-statistic on the coefficient of the time variable.

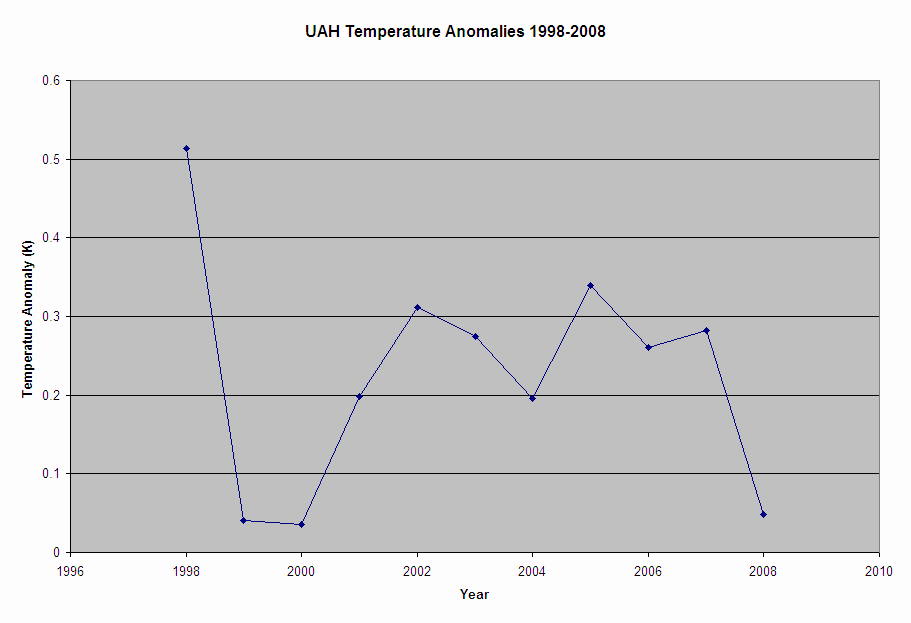
Let's get quantitative. Here are the UAH annual temperature anomalies for 1998-2008:

Here's a chart of the data. Dramatic, isn't it?

If we regress anomaly on year, we get the following regression equation:

Anom = 9.733 - 0.000475 Year

The t-statistic on the Year term is -0.32, meaning the slope is statistically indistinguishable from zero and there is no way to tell if the actual trend is up or down. Again, see point 1. You need an adequate sample size, and VV hasn't got one.

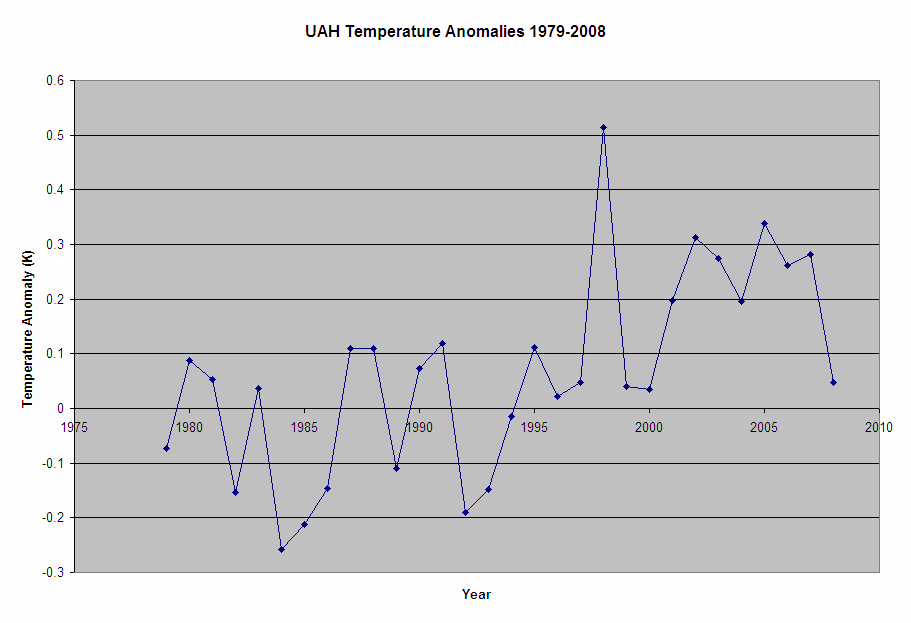
Now, let's do the analysis over with an adequate sample size. The WMO recommends 30 years. Here are the UAH anomalies for the last 30 years:

And here's the chart for this data. Notice how VV picked out just the right-hand portion, starting from the obvious outlier of 1998 (the largest El Nino on record), to get his "downward trend:"

Now the regression equation is:

Anom = -25.265 + 0.0127 Year

And the t-statistic on the year term is 4.13, significant at well beyond the 99% confidence level. The temperature is rising, the increase is statistically significant. So says the UAH satellite record.

What does all this tell us?

1. Don't cherry-pick your start point to make the answer come out the way you want.
2. Use an adequate sample size.
3. Make sure the slope you're talking about is statistically significant.

For more on time series analysis, I highly recommend a book like Gujarati's "Basic Econometrics" (1978). But any introductory statistics textbook should have a section on regression analysis and significance testing.